Recently, I have been helping a third grade teacher learn how to use Number Talks to  develop computational fluency and deepen student understanding of multiplication and division.  The first time we met, we planned a Number Talk using MiniLessons for Early Multiplication and Division by Willem Uttenbogaard and Catherine Twomey Fosnot.  We rehearsed the Number Talk.  As we discussed the math, we anticipated what students might say, how Rachel might record student thinking, and what questions she might ask to connect student thinking. We read the teacher’s guide and noticed some of our ideas in the description. We discussed how Rachel could follow the same structure we just used to plan subsequent Number Talks.

Last Thursday, Rachel and I met again to discuss how the number talk went. She told me she was so glad I showed her how to use her Smartboard for Number Talks.  She described how helpful it was for her students to be able to describe their own thinking and see each other’s thinking. She pulled up the Smartboard Notebook she had created for the last Number Talk so we could discuss her student’s thinking. Unfortunately, during this Number Talk, the markers on her Smartboard weren’t working so she had to record student thinking with numbers, symbols, and words.

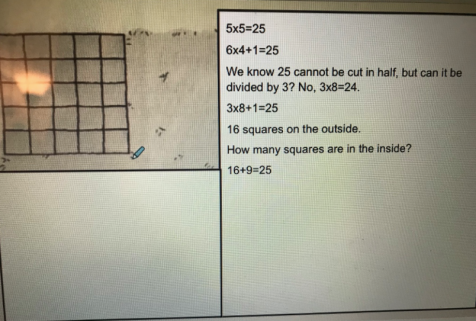
At this point, Rachel asked me an awesome question.  She wondered, “my students started doing this thing where they find a fact that doesn’t work evenly and then add the extra squares. Should I encourage them to do that? Will it distract them for what they should be doing?” I asked her to tell me more. She showed me some examples. She said the students were thinking about division. They were noticing that you couldn’t divide 25 in half. They tried to divide it into three groups. One of the students said, “You can do it any way you want. You just have to add the extras.”

Rachel looked at me, waiting for me to tell her what to do next.

She said, “Should I let them do this?”

I responded, “I don’t know. Let’s find out.”

Then, I got really excited.  “Oh my gosh, are they thinking about division? Are they thinking about remainders, but they don’t know it.  This is so cool. Do they wonder if this will always work? Do they wonder how many ways there are? I’m wondering how many ways there are! Are there a certain number of ways? Does it depend on the array? What does this have to do with factors? There must be a pattern. Is there a pattern?  Can we do the math? Do you want to explore this right now? I feel like we have to explore this right now to answer your question? Are you okay if we try this right now?”

Rachel smiled. I started scribbling things down on scrap paper.

It didn’t take long before we realized we needed some kind of system for organizing our thinking.

At this point, we started talking about the commutative property.  We discussed the difference between 3 groups of 8 and 8 groups of 3. We wondered, “are we only using arrays or can we use “groups”?” If we are only using arrays than we can’t see any 3 by 8 arrays in a 5×5 array. We can see seven 3 by 1 arrays, but then we have 4 leftovers. We can’t make anymore 3 by 1 arrays. If we are using groups, we can see 8 groups of 3 plus one leftover and we can also see 3 groups of 8 plus one left over. We were in this funny place. We were mixing array language with “equal groups of” language, trying to figure out how the constraints of contextualizing the community property impacted our problem.  I thought about this some more this weekend. I tried to anticipate what students might do when they confront this situation.

There is the potential for this conversation to get messy fast. We could all end up on the fast track down a major rabbit hole. I think it is worth the risk.  As I explored this problem, I was forced to articulate the difference between an area representation of multiplication and a set representation of multiplication.  These third grade students are about to start their unit on area and perimeter. This seems like a meaningful mess.  It might be worthwhile to pause during the exploration, examine a few of the decompositions and discuss; What is the same? What is different?

When Rachel and I were exploring this problem, we didn’t define the constraints. We were in the initial stages of our problem solving. We were messing around with  mathematics. We were thinking about arrays, but using “groups of” language. Towards the end of our exploration, Rachel stumbled upon a conjecture. She said, “I think we have found all the ways, but I can’t really explain why.” I asked her to tell me what she was thinking, even if it was still fuzzy. I wrote down what she said:

She said she wasn’t sure how to describe what needed to be bigger, but she just knew that we could make more groups with the leftovers. I knew what she meant, but I couldn’t find the precise words for it, either. I tried to use numbers and symbols to record what was happening:

 

Then, Rachel said, “I think I can explain why we can’t find any more ways. If the number of leftovers is larger than the group size, you will always be able to make more groups.”

“Yes. That makes sense to me.”

While Rachel and I were working, every so often, we would zoom out and discuss the implications of Rachel exploring this problem with her students. She wondered, would it confuse them? Would it dissuade them from using efficient strategies? I kept trying to find connections to the third grade standards.  I was reminded of an excerpt from Bill McCallum’s progressions document:

I saw a lot of connections to fourth grade standards, particularly the one about interpreting remainders. Rachel and I both worried, “is it okay if these students start talking about remainders if they are only in third grade?”

I have been thinking about this 4th grade standard a lot this year.  Marilyn Burns, Kristin Gray, and Jody Guarino have all pushed my thinking on this standard. I started a post about a lesson I did with a fourth grade class earlier in the year. I haven’t finished it, but I’m posting it in draft form because it is really connected to this post. How is this fourth grade standard connected to work in third grade? It should be, right? Mathematics is a system of interconnected ideas. Remainders don’t just drop out of the sky in fourth grade? They shouldn’t, right? What might we explore in third grade that would connect to a deeper exploration of remainders in fourth grade? I’m thinking about this cluster:

I’m not totally sure if/how these standards might connect to the problem that Rachel is  going to explore with her students, but I’m wondering about it.

I shared some of these thoughts with Rachel. Together, we decided this problem would be an opportunity to discuss the difference between arrays and groups, connect multiplication and division, look for structure, persevere, and organize thinking. Should Rachel create a space for her students to explore this problem?

Of course she should! They created it!  It is their problem!

We talked about how she would present the problem and support the student exploration. We revisited how the question originally came about and how she would phrase the question so it was clear and still captured the student’s voice.

Rachel took all of our work with her so she could share it with her students after they tackled the problem. She thought they would be really excited to see that we worked so hard on a problem that they created.  I’m still thinking about ways that Rachel can use this problem as a spring board to deepen understanding of grade level standards.  She plans on using it with her students this coming Monday. I would love to hear your thoughts about questions Rachel could ask, connections she might make, next steps for her students.  Try this problem out! Get messy! Let us know what you find out.